Wasim Akram vs Dennis Lillee

[Migara]

 

[Burgey]

I ran the stats through the same variables Migara did and McGrath's adjusted average is 3.600000004 and Wasim's 3.6643.

McGrath therefore miles better.

 

[Migara]

I ran the stats through the same variables Migara did and McGrath's adjusted average is 3.600000004 and Wasim's 3.6643.

McGrath therefore miles better.

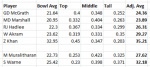
The difference between McGrath and Wasim widens when adjusted.

To get better idea, I think WPI should be included as well.

 

[TheJediBrah]

can't believe Migara actually did it

 

[_00_deathscar]

I was just about to ask when we get to Warne vs Murali.

 

[Pap Finn Keighl]

View attachment 29915

Please explain the method, if you are serious.

 

[TheJediBrah]

Please explain the method, if you are serious.

Self explanatory if you read his last couple posts

 

[Gob]

I want to know too

 

[Migara]

Please explain the method, if you are serious.

Fractions of
Top order wickets - x
Middle order - y
Lower order - z

Relative weight of top order wicket 1.225, middle order wicket 1.159, lower order wicket 0.505 (base on averages)

bowling average a, adjusted average A

A = ax/1.225 + ay/1.159 + cz / 0.505

You may even look at A/a or A - a as well.

 

[Kirkut]

You can add diabetes...

Imran had all the above challenges plus a 2-year shin injury to recover from.

Like most ATG pace bowlers, Akram had three phases to his career: early development (85-89), peak (90-97) and then late career dip (98-2002). During his peak, he, along with Ambrose, was generally considered the best bowler in the world. The problem is that his early development and late career were unusually longer for an ATG bowler, counting for more than half of his career.

For example, McGrath, and Hadlee basically had a very minor dip towards the end. Ambrose and Marshall had slightly long dips but still averaged well despite taking less wickets. Imran like Wasim also had long early and late career phases, but his peak was much better than virtually any bowler. Lillee was almost equally prolific in pretty much every stage of his career. Steyn had a very short early career phase and went very quickly into his long peak.

Imran wasn't diabetic, plus from the footage I've watched from the 80s, he got way too many easy lbws. Wasim and Waqar had their yorkers on point, so no need for qualified umpiring here.

 

[Kirkut]

Among all the elite ATG bowlers (Hadlee aside) Akram had the biggest challenges to overcome. Controversies, below par fielders, bowling from the junk end, burden of captaincy, extra long career, undisciplined team ( if we can call so ), unfavorable conditions... Nothing stopped him form being the best bowler in the world though.

No, he's a far superior bowler in the subcontinent than in Australia or South Africa.

 

[ankitj]

Fractions of
Top order wickets - x
Middle order - y
Lower order - z

Relative weight of top order wicket 1.225, middle order wicket 1.159, lower order wicket 0.505 (base on averages)

bowling average a, adjusted average A

A = ax/1.225 + ay/1.159 + cz / 0.505

You may even look at A/a or A - a as well.

That doesn't make sense. Treating all top order batsman as having same average.

 

[TheJediBrah]

That doesn't make sense. Treating all top order batsman as having same average.

It's a generalisation but it makes perfect sense. If you want to go and count the average of the batsman of every single wicket they took and average them all out then go for it. This would be cool af.

 

[trundler]

It's a generalisation but it makes perfect sense. If you want to go and count the average of the batsman of every single wicket they took and average them all out then go for it. This would be cool af.

Ankit literally did that though.

How valuable is that wicket!

Long time back I came across a comprehensive analysis of bowlers based on the "value"of the wickets they take. The approach was wonderfully elegant and very logical. The article is not online anymore. Nevertheless I decided to do the exercise again. I am happy to share the results of my hard...

www.cricketweb.net

 

[TheJediBrah]

Ankit literally did that though.

How valuable is that wicket!

Long time back I came across a comprehensive analysis of bowlers based on the "value"of the wickets they take. The approach was wonderfully elegant and very logical. The article is not online anymore. Nevertheless I decided to do the exercise again. I am happy to share the results of my hard...

www.cricketweb.net

Wow that's great. I wasn't aware. Looks like pretty much the same results as Migaras mini-effort.

With the exception of Warne v Murali.
Warne actually has a higher average value of wicket. This could be explained by Murali playing so much against Bang and Zim so doesn't really tell you much

 

[Migara]

Wow that's great. I wasn't aware. Looks like pretty much the same results as Migaras mini-effort.

With the exception of Warne v Murali.
Warne actually has a higher average value of wicket. This could be explained by Murali playing so much against Bang and Zim so doesn't really tell you much

Ideally you have to generalize the number of matches each player against each team. Say if their are 9 sides we have to assume as 12.5% of matches played against each opposition for the calculations to be meaningful. There may be a bit of error when a team enters the arena in the middle of a particular player's career though.

 

[TheJediBrah]

Ideally you have to generalize the number of matches each player against each team. Say if their are 9 sides we have to assume as 12.5% of matches played against each opposition for the calculations to be meaningful.

Why on earth would you do that? It would just make the results meaningless and fantasy

It's a pretty perfect representation the way it is

 

[Migara]

Why on earth would you do that? It would just make the results meaningless and fantasy

It's a pretty perfect representation the way it is

That would even out the effect of some teams playing more head to head games.

 

[TheJediBrah]

That would even out the effect of some teams playing more head to head games.

That makes no sense. The different oppositions is part of why the results are different. If you "even out" that effect you're making meaningless fantasy stats that didn't happen.

If you're saying that to make Murali look better it's not necessary. The fact that his average value per wicket is only 1 run worse than Warne's yet his career average is 4 runs lower already puts things in his favour.

 

[Migara]

That makes no sense. The different oppositions is part of why the results are different. If you "even out" that effect you're making meaningless fantasy stats that didn't happen.

That makes perfect sense. If a player keeps bashing two or three oppositions in 75% of his matches and gets walloped greater number of oopsitions in other 25%, the overall stats are hugely warped than a player who does well against every team but has higher overall average.